|
Welcome to the research group
Differential Geometry and Geometric Structures
|
|
Members & friends of the group in Jul 2021
photograph © by Narges Lali
|
Differential geometry has been a thriving area of research
for more than 200 years, employing methods from analysis
to investigate geometric problems. Typical questions
involve the shape of smooth curves and surfaces and the
geometry of manifolds and Lie groups. The field is at
the core of theoretical physics and plays an important
role in applications to engineering and design.
Finite and infinite geometric structures are ubiquitous
in mathematics. Their investigation is often intimately
related to other areas, such as algebra, combinatorics or
computer science.
These two aspects of geometric research stimulate and
inform each other, for example, in the area of "discrete
differential geometry", which is particularly well suited
for computer aided shape design.
|
|
Gallery of some research interests and projects
Symmetry breaking in geometry:
We discuss a geometric mechanism that may,
in analogy to similar notions in physics,
be considered as "symmetry breaking" in geometry.
(Fuchs, Hertrich-Jeromin, Pember;
Fig ©Nimmervoll)
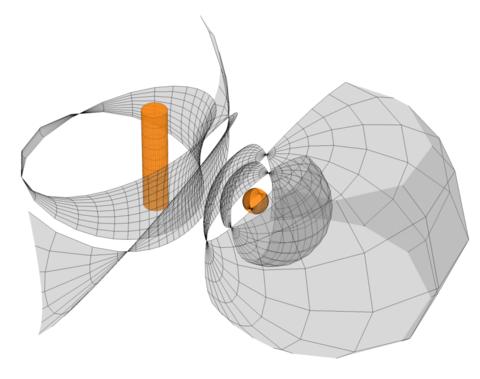
Cyclic coordinate systems:
an integrable discretization in terms of a discrete flat
connection is discussed.
Examples include systems with discrete flat fronts or
with Dupin cyclides as coordinate surfaces
(Hertrich-Jeromin, Szewieczek)
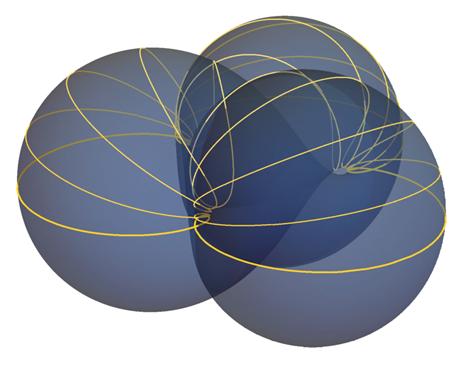
We study surfaces with a family of
spherical curvature lines
by evolving an initial spherical curve through
Lie sphere transformations,
e.g., the Wente torus
(Cho, Pember, Szewieczek)
Discrete Weierstrass-type representations
are known for a wide variety of discrete surfaces classes.
In this project, we describe them in a unified manner,
in terms of the Omega-dual transformation applied to
to a prescribed Gauss map.
(Pember, Polly, Yasumoto)
Billiards:
The research addresses invariants of trajectories of a
mass point in an ellipse with ideal physical reflections
in the boundary. Henrici's flexible hyperboloid paves the
way to transitions between isometric billiards in ellipses
and ellipsoids (Stachel).
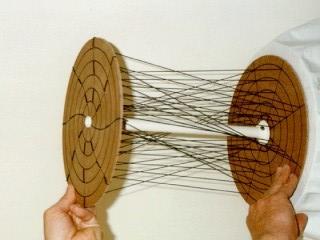
Spreads and Parallelisms:
The topic of our research are connections among spreads
and parallelisms of projective spaces with areas like
the geometry of field extensions, topological geometry,
kinematic spaces, translation planes or flocks of quadrics.
(Havlicek)
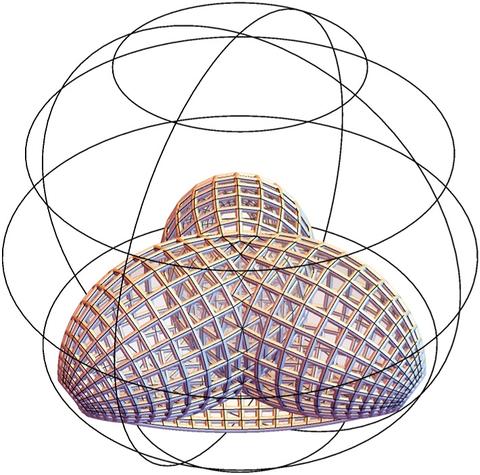
This is a surface of (hyperbolic) rotation in hyperbolic
space that has constant Gauss curvature,
a
recent classification project.
(Hertrich-Jeromin, Pember, Polly)
Geometric shape generation:
We aim to understand geometric methods to generate
and design (geometric) shapes,
e.g., shape generation by means of representation formulae,
by transformations, kinematic generation methods, etc.
(Hertrich-Jeromin, Fig Lara Miro)
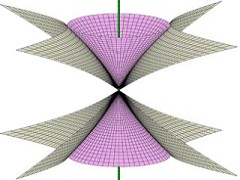
Affine Differential Geometry:
In affine differential geometry a main point of research is
the investigation of special surfaces in three dimensional
affine space.
(Manhart)
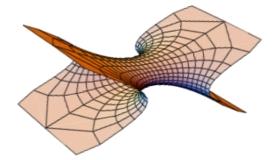
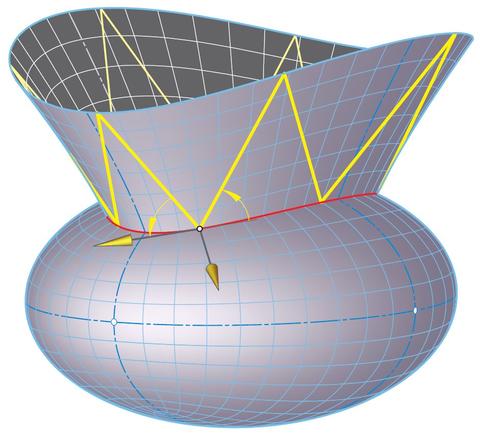
Transformations & Singularities:
We aim to understand how transformations of particular
surfaces behave (or fail to behave) at singularities, and
to study how those transformations create (or annihilate)
singularities.
The figure shows the isothermic dual of an ellipsoid,
which is an affine image of a minimal
Scherk tower.
(Hertrich-Jeromin)
|
|
News
- 27 Jun 2024: Geometry seminar
- Ilya Kossovskiy (Masaryk University in Brno and TU Wien):
TBA
- 20 Jun 2024: Geometry seminar
- Denis Polly (TU Wien):
TBA
Abstract
...
- 13 Jun 2024: Geometry seminar
- Fabian Achammer (TU Wien):
TBA
Abstract
...
- 06 Jun 2024: Geometry seminar
- Kiumars Sharifmoghaddam (TU Wien):
TBA
Abstract
...
- 23 May 2024: Geometry seminar
- Georg Nawratil (TU Wien):
TBA
Abstract
...
- 16 May 2024: Geometry seminar
- Martina Iannella (TU Wien): TBA
- 02 May 2024: Geometry seminar
- Niklas Affolter (TU Wien):
TBA
Abstract
...
- 25 Apr 2024: Geometry seminar
- Alessandro Andretta (University of Turin):
The Banach-Tarski paradox
Abstract
One of the most surprising results of modern mathematics is the
following result proved by Hausdorff, Banach and Tarski:
the unit ball of the euclidean space can be partitioned in a
finite number of pieces so that these can be rearranged, using
rigid motions so to form two balls identical to the original.
The proof is non-constructive, relying on the Axiom of Choice,
and the pieces of the decomposition are inconceivably sharp and
edgy!
Geometry plays a substantial role, as the core of the proof is
based on the existence of a free subgroup of the group of
rotations.
(A similar result cannot be proved for the plane, i.e. it is not
possible to duplicate a disk.)
In this talk I will sketch the proof of the Banach-Tarski
paradox, and survey many related results that have been proved
in the following years.
18 Apr 2024: Geometry seminar - Ivan Izmestiev (TU Wien): Cayley-Bacharach theorem and sums of squares
Abstract
The Cayley-Bacharach theorem (first proved by Chasles) says that
if two cubics meet at nine points, then any other cubic passing
through eight of these nine points also passes through the
ninth. This theorem includes as special cases the Pappus and the
Pascal theorems.
The sums of squares problem was posed by Hilbert: can every
positive definite homogeneous polynomial of degree $2d$ in n
variables be represented as a sum of squares of polynomials of
degree $d$? While the answer is positive for $d=1$ and n arbitrary
as well as for d arbitrary and $n=2$, Hilbert has proved the
negative for $d=3$ and $n=3$. And a crucial point in his proof was
the Cayley-Bacharach theorem.
This talks is based on the articles by Eisenbud-Green-Harris and
Blekherman.
- 21 Mar 2024: Geometry seminar
- Gudrun Szewieczek (TU Munich):
Discrete isothermic nets with a family of spherical parameter lines from holomorphic maps
Abstract
Smooth surfaces with a family of planar or spherical curvature
lines are an active area of research, driven by both purely
differential geometric aspects and practical applications such
as architectural design. In integrable geometry it is a natural
question to ask which of these surfaces admit a conformal
curvature line parametrization and are therefore isothermic
surfaces.
It is an open problem to explicitly describe all those smooth
isothermic surfaces. However, over time, prominent examples were
found in this rich integrable surface class: above all Wente's
torus. More recently, further specific examples have led to the
discovery of compact Bonnet pairs and to free boundary solutions
for minimal and CMC-surfaces.
This talk covers a discrete version of the problem: we shall
generate all discrete isothermic nets with a family of spherical
curvature lines from special discrete holomorphic maps via the
concept of "lifted-folding".
In particular, we point out how this novel approach leads to
quasi-periodic solutions and to topological tori with
symmetries.
This is joint work with Tim Hoffmann.
- 14 Mar 2024: Geometry seminar (Sem.R. DB gelb 03)
- David Sykes (TU Wien):
CR Hypersurface Geometry, an Introduction
Abstract
CR geometry concerns structures on real submanifolds in complex
spaces that are preserved under biholomorphisms. This talk will
present a light introduction to CR geometry of real
hypersurfaces. We will survey some of the area's major
classical results, namely solutions to local equivalence
problems of E Cartan, Tanaka, and Chern-Moser and their
applications. And we will preview some of the area's current-day
research trends related to Levi degenerate structures.
|
Technische Universität Wien
